Numerical simulation of fluid-structure interaction problems

The analysis of fluid–structure interaction (FSI) problems, in which the structure undergoes large deformations and the fluid motion is characterized by free surfaces and breaking waves, is of great relevance in many areas of engineering.

A possibility to overcome the difficulties related to the tracking of the interfaces between fluids and solids is the use of a Lagrangian approach for both the fluid and the solid parts.

We proposed a (FSI) approach in which the fluid si described with the Particle Finite Element Method (PFEM) and the structure with the Finite Element Method. Tha Lagrangian nature of the PFEM, toghether with its efficient mesh management allows for the automatic tracking of the free surfaces.

Fully explicit approach for FSI with nonconforming meshes and different time step size

We recently proposed a fully explicit partitioned method for the simulation of Fluid Structure Interaction (FSI) problems. The fluid domain is modelled with an explicit Particle Finite Element Method (PFEM) based on the hypothesis of weak compressibility. The Lagrangian description of the fluid is particularly effective in the simulation of FSI problems with free surface flows and large structural displacements,  since the fluid boundaries are automatically defined by the position of the mesh nodes. The  solid domain is modelled using the explicit integration FEM.

The structure-to-fluid coupling algorithm is based on a technique derived from the Domain Decomposition Methods, namely, the Gravouil and Combescure algorithm. The method allows for arbitrarily large interface displacements using different time incrementation and nonconforming meshes in the different domains, which is an essential feature for the efficiency of an explicit solver involving different materials.

The resulting fully explicit and fully lagrangian finite element approach is particularly appealing for the possibility of its efficient application in a large variety of highly non-linear engineering problems

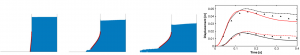
Figure: opening of an elastic gate. In the graph, dots represents experimental data and black lines are the results of the proposed approach.

Meduri S., Cremonesi M., Perego U., Bettinotti O., Kurkchubasche A., Oancea V. (2018), A partitioned fully explicit Lagrangian Finite Element Method for highly nonlinear Fluid-Structure-Interaction problems, International Journal for Numerical Methods in Engineering, vol 113(1), pp. 43-64, DOI:10.1002/nme.5602

Simulation of airbag deployment

An airbag is a passive automotive safety system built into the steering wheel and other strategic locations of a vehicle. In the case of accidents, these inflatable cushions restrain automobile passengers dissipating their kinetic energy and thereby reducing the risk of injury. The design of inflatable restraint system is an engineering  problem of great challenges.

Flat airbag deployment.

A full FSI simulation of the airbag deployment is presented. Due to the extremely fast dynamics and high degree of non-linearity, explicit dynamics solvers will be privileged. Moreover, this phenomenon shows a strong evolution of the interface between the fluid and the structure. The airbag is initially empty and folded inside the device housing and then the fluid flow causes the complex deployment until the completely in ated configuration. Such strong variations of the fluid structure interface can be naturally tracked by the fully Lagrangian kinematics. Finally, a partitioned approach is preferable, since the large number of complex modelling aspects both on the fluid and structural sides would lead to several issues in the implementation of a unique monolithic solver.

Flat airbag deployment against spherical mass.

Meduri S., Cremonesi M., Frangi A., Perego U. (2022) A fluid-structure interaction approach for the simulation of airbag deployment, Finite Elements in Analysis and Design, Vol. 198, 103659, DOI:10.1016/j.finel.2021.103659